Bài 2 trang 91 SGK Hình học 11

Đề bài

Cho hình hộp \(ABCD.A'B'C'D'\). Chứng minh rằng:

a) \(\overrightarrow{AB}\) + \(\overrightarrow{B'C'}\) + \(\overrightarrow{DD'}\) = \(\overrightarrow{AC'}\);

b)  \(\overrightarrow{BD}\) - \(\overrightarrow{D'D}\) - \(\overrightarrow{B'D'}\) = \(\overrightarrow{BB'}\);

c)  \(\overrightarrow{AC}\) + \(\overrightarrow{BA'}\) + \(\overrightarrow{DB}\) + \(\overrightarrow{C'D}\) = \(\overrightarrow{0}\).

Hướng dẫn giải

Dựa vào các vector bằng nhau và quy tắc ba điểm.

Lời giải chi tiết

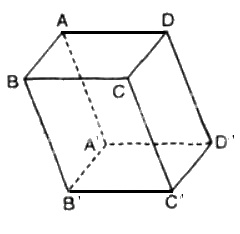
a) \(\overrightarrow{AB}\) + \(\overrightarrow{B'C'}\) + \(\overrightarrow{DD'}\)  = \(\overrightarrow{AB}\) + \(\overrightarrow{BC}\) + \(\overrightarrow{CC'}\) = \(\overrightarrow{AC'}\); 

b) \(\overrightarrow{BD}\) - \(\overrightarrow{D'D}\) - \(\overrightarrow{B'D'}\) = \(\overrightarrow{BD}\) + \(\overrightarrow{DD'}\) + \(\overrightarrow{D'B'}\) = \(\overrightarrow{BB'}\);

c) \(\overrightarrow{AC}\) + \(\overrightarrow{BA'}\) + \(\overrightarrow{DB}\) + \(\overrightarrow{C'D}\) = \(\overrightarrow{AC}\) + \(\overrightarrow{CD'}\) + \(\overrightarrow{D'B'}\) + \(\overrightarrow{B'A}\) = \(\overrightarrow{0}\).