Bài 6 trang 12 SGK Hình học 10

Đề bài

Cho hình bình hành $ABCD$ có tâm $O$. Chứng minh rằng:

a) $\overrightarrow{CO} - \overrightarrow{OB} = \overrightarrow{BA}$;

b) $\overrightarrow{AB} - \overrightarrow{BC} = \overrightarrow{DB}$;

c) $\overrightarrow{DA}  -\overrightarrow{DB} = \overrightarrow{OD} - \overrightarrow{OC}$;

d) $\overrightarrow{DA} - \overrightarrow{DB} + \overrightarrow{DC} = \overrightarrow{0}$.

Hướng dẫn giải

Với quy tắc ba điểm tùy ý $A, \, \, B, \, \, C$ ta luôn có:

$+ )\;\overrightarrow {AB}  + \overrightarrow {BC}  = \overrightarrow {AC}$ (quy tắc ba điểm).

$+ )\;\overrightarrow {AB}  - \overrightarrow {AC}  = \overrightarrow {CB}$ (quy tắc trừ).

Lời giải chi tiết

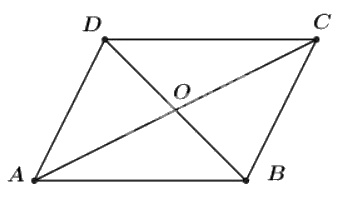
a) Ta có: $\overrightarrow {CO}  = \overrightarrow {OA}$$\Rightarrow \overrightarrow {CO}  - \overrightarrow {OB}  = \overrightarrow {OA}  - \overrightarrow {OB}  = \overrightarrow {BA} .$

b) Ta có: $\overrightarrow {BC}  = \overrightarrow {AD}$$\Rightarrow \overrightarrow {AB}  - \overrightarrow {BC}  = \overrightarrow {AB}  - \overrightarrow {AD}  = \overrightarrow {DB} .$ 

c) Ta có : $\left\{ \begin{array}{l} \overrightarrow {DA} - \overrightarrow {DB} = \overrightarrow {BA} \\ \overrightarrow {OD} - \overrightarrow {OC} = \overrightarrow {CD} \end{array} \right..$

Mà $\overrightarrow {BA}  = \overrightarrow {CD}$$\Rightarrow \overrightarrow {DA}  - \overrightarrow {DB}  = \overrightarrow {OD}  - \overrightarrow {OC} .$

d) Ta có: $\overrightarrow {DA}  - \overrightarrow {DB}  + \overrightarrow {DC}  = \overrightarrow {BA}  + \overrightarrow {DC}$$= \overrightarrow {BA}  + \overrightarrow {AB}  = \overrightarrow 0 .$